
I put my youngest image here because the groundwork of what I offer belongs to 1965 when I first thought about the problem and 1968 when my paper about it was published. I should have put in as a bookend my latest and oldest image, too, for I completed the thought just last summer.
The thought is about clearance, the very foundation of all renal physiology, and how I have always had it wrong, or perhaps better said, had an incomplete view of it.
Probably most of us have done just fine with that incomplete view.
But I mean to show you that traditional clearance and its practical uses are a limited version of what nature has to tell us. I think the larger story is beautiful, if a bit abstract, and probably has practical value, too.
The shapely equation is from my earliest work, and gives rise to that larger story I want to tell you.
Why Should Stone Formers Care?
Measured Kidney Function Gives Part of the Story
I will show you, when I have finished, how even small changes in glomerular filtration – what physicians can measure in their patients – may cause large effects on the body.
Clearance relates urine excretion rates to plasma levels of things. Everyone knows this. But, although we do not usually speak about the matter, clearance also gauges how long it takes for things made or eaten to pass through the body. Therefore fall in clearance may raise both the concentrations of undesirable molecules we make or eat and also their dwell times in the body creating a much larger exposure of cells to them.
That term, exposure, is perhaps best stated as the product of the concentration of such a molecule multiplied by how long it is present: the concentration – time integral. This can be reduced to the simple idea of the average concentration (mean concentration) times the average time it is present (mean time).
Stone Formers Have Modest Reductions in Kidney Function
Forming stones raises risk of reduced kidney function and of seemingly unrelated problems like hypertension, stroke, heart attack, and bone fractures. Possibly the hidden effects of reduced clearance – longer dwell times for molecules we eat or produce from food – could link these increased risks together.
That is conjecture, but sometimes we should pause and look at all the alternatives, physicians, scientists, and patients alike.
What Follows Only Looks Difficult – it is not
I use equations, but they say obvious things no more complex than in accounting and a lot easier than compound interest. The underlying derivation of the work did cost me some serious effort long ago, but I do not show it here, simply use the answer I got.
Math phobes, be brave and challenge yourselves.
Math lovers, there is the original paper. Derive everything yourselves. Offer me better ways. I am not a mathematician, just a journeyman scientist using whatever tools seem fit to hand.
Traditional Clearance
No matter what the material is, if it is in the blood and removed by the kidneys into the urine we can always calculate its ‘clearance’.
It goes this way.
How To Calculate Clearance
We Clear From Plasma Into Urine
Whatever is in the urine, assuming the kidneys do not make it and put it into the urine directly from its site of production, came from the blood. In particular it comes from the blood plasma, as the red blood cells are not carrying most molecules in a form fit to renal processing. Because of common practice, it is usually serum (what is leftover after clotting) we measure materials in. But in life it is blood plasma, so I will refer to it henceforth.
We Need To State an Equation
Perhaps the material was filtered by the renal glomeruli, perhaps the tubule cells transported it out of the plasma into the tubule fluid and thence it traveled to the urine, perhaps both. But if you know the plasma concentration and the rate at which the material is appearing in the urine, you can always calculate its urinary or external ‘Clearance‘:
Urine removal rate = plasma concentration times ‘clearance’.
We Need To Formalize the Equation
If you cannot say it in words, you do not understand the idea. So what I just wrote is very important as proof of understanding.
If you cannot say it in mathematics, you cannot use the idea.
Lets call ‘i’ the name of something whose clearance we want to calculate. Lets bold equations and their terms in the text. Lets re-state what I just said in words:
Eq. 1a: Ui x Vu = Pi x Ci
where Ui is the urine concentration of i (mass/volume), Vu is urine flow rate (volume/time) and Pi is the plasma concentration of i (mass/volume).
Now, lets isolate clearance on one side of the equal sign, and put the rest – the actual things we measure – on the other side:
Eq. 1b: Ci = (Ui x Vu) / Pi
This makes Ci the ratio of concentration x (volume / time) / concentration, and because the units of concentrations on the top and bottom of the division sign cancel out, we are left with just volume / time. So clearance has the units of a flow rate.
Looked at another way, clearance is the relationship between the traffic of i through the body (Ui x Vu) and its plasma concentration, Pi .
Physicians all use clearance. Patients hear about their creatinine clearance as a measure of their glomerular filtration rate. Everyone accepts clearance as an important measurement, and they are right to do so. But you must admit clearance has some mysterious properties: a flow rate, and a relationship between traffic and concentration. .
Eventually we have to figure out what it means to be a flow rate and also a relationship between plasma traffic and concentration.
Restrictions on Clearance
If the kidneys make i and put all they make into the blood plasma, the kidneys are identical to any other source of the material and the clearance equation holds.
If the kidneys metabolize or otherwise dispose of i so it does not reach the urine, they are like any other part of the body cell mass, and external, or urinary clearance still holds. It holds because clearance of i into the urine remains unaltered even if the kidney cells ‘clear’ i partly by metabolizing it.
But, If the kidneys make i and put it into the urine directly from its site of kidney production without passing what they made through the blood, clearance calculated from urine no longer holds. The amount of i in the urine does not arise entirely from plasma, but from plasma and kidney cells combined.
Clearance is a Conservation Statement
Clearance is to say that i does not appear in the urine except from plasma. We know how much i is coming into the urine in any given time, so there must be some factor that will convert the plasma concentration of i into the amount of i coming into the urine, and we call that factor the clearance. That is why the kidneys cannot make i and put it into the urine without passing it through the blood plasma, but why kidneys can make i and put it into the urine via the blood plasma.
The Units of Clearance
I have said that clearance has the units of volume per unit time, but I have not said what that means in real, practical terms. What volume? What time? Why a volume per unit time in the first place?
Clearance Taken Literally
There is only one way to make that volume/time mean anything as a general rule.
Because clearance is based on strict conservation, the plasma has to provide what is coming into the urine on an ongoing rate – plasma is the only source of the urine material.
Therefore, clearance is the volume of plasma whose content of i could provide all the i that enters the urine during some time period*.
Put another way, the material is constantly appearing in the urine, plasma is its source, the kidneys are removing it into the urine, and clearance gives the plasma volume one could calculate with the assumption that all of the material was removed.
*Things get icky here as removal is estimated from plasma but in some cases the total blood flow is calculated using the volume percent of red blood cells. Sometimes red blood cells might carry some cleared molecules. I mean to pass this by as being unneeded right now.
Clearance Taken with a Dose of Reality
In fact, that notion of clearance is virtually never true. Kidneys almost never extract all of anything from the blood plasma that flows through them.
So clearance is not taken literally except in a few special instances.
Is this not a strange reality? Conservation is perfect, undeniable, yet it gives us a calculation at remarkable distance from what kidneys usually do.
Practical Uses of Clearance
In a few special situations clearance quantifies events that really happen, and we use it to gain practical insights. It is these few situations that have elevated conservation based clearance calculations into a near immortality, made them the central core, the ruling engine of renal medicine and renal physiology.
Glomerular Filtration
I have already detailed the most common and important use of clearance, to measure glomerular filtration.
For clearance to gauge filtration, the substance i must be freely filtered by the glomeruli and neither taken back up into the blood nor secreted out of blood by the kidney tubule cells, nor metabolized by the kidney, nor – as holds for all urine clearances – made in kidneys and delivered directly into urine without passing through the blood.
Few substances satisfy all these restrictions. But perfect markers do exist, and upon them generations have based the huge edifice of renal function.
The most common marker we use in medicine, creatinine, is poor indeed.
Even so, glomerular filtration calculated from creatinine undergirds clinical renal medicine, even all medicine altogether, as filtration seems most directly connected to survival. So important is clearance, and so common to all of medicine, few have paid much attention to how narrow a base conservation affords to clearance, and how much about clearance it cannot reveal.
Fractional Reabsorption and Secretion
Filtered Load
Once we know glomerular filtration, we can calculate the amount of something filtered by the glomeruli – the filtered load.
It is simply the product of glomerular filtration rate (GFR) times the concentration of that something in plasma that is free to filter – not bound to molecules too large to pass through the filtration system. Often we make filtrates from blood plasma to measure the ‘ultra-filterable’ concentration of what we are interested in.
Fraction Excreted
Given a urine loss rate and a filtered load, we can form their fraction: Urine excretion/Filtered load.
That fraction is the fraction of filtered material let pass through into the urine. If it is above 1, then the material must be secreted from plasma into urine – always assuming kidneys do not produce it and send it into urine without its passing through the circulation. If it is below 1 then the tubules are reabsorbing the material – assuming the kidneys do not metabolize if from the tubule fluid.
Tubule handling of myriads of molecules gave rise to modern human kidney physiology, and physicians use such calculations in their daily practice of renal medicine. So, like GFR, they are not just commonplace but the bedrock of a whole branch of medicine and science.
Renal Blood Flow
A few molecules are filtered and also secreted from plasma into tubule fluid with such vigor that almost all is removed during a single passage of plasma through the kidneys. Therefore their clearances are close to the volume of plasma passing through the kidneys. If we account for the volume of blood occupied by red blood cells, and make allowances when necessary for carriage of the material in red blood cells, we can estimate renal blood flow. This has been done for decades.
Clearance as a Kinetic Parameter of an Open System
If you view the kidneys not in isolation but as part of a system that includes entry into blood plasma as well as removal from it, clearance does not depend at all on conservation. It arises naturally, from the inherent properties of a system open at both ends – food and fluids going into blood plasma, stuff leaving from blood plasma into the urine. In an open system, clearance estimates how long it takes for a bolus of something (nutrient or toxin) to pass through.
The Open System
We – and all animals with kidneys – live in a quasi steady state. We eat, we drink, stuff comes into plasma in boluses that depend on our habits and and how the gut regulates things.
Kidneys remove stuff from plasma as it enters, sometimes regulating to keep interior concentrations of certain things very constant (calcium, for example) and sometimes just ushering things out – lots of drugs, metabolites – as fast as possible.
As Fast as Possible
That phrase gives a clue. Kidneys remove things, but from a system with a volume of plasma – and more – to process from. Clearance gives the kinetics of removal, how long things dwell after entering.
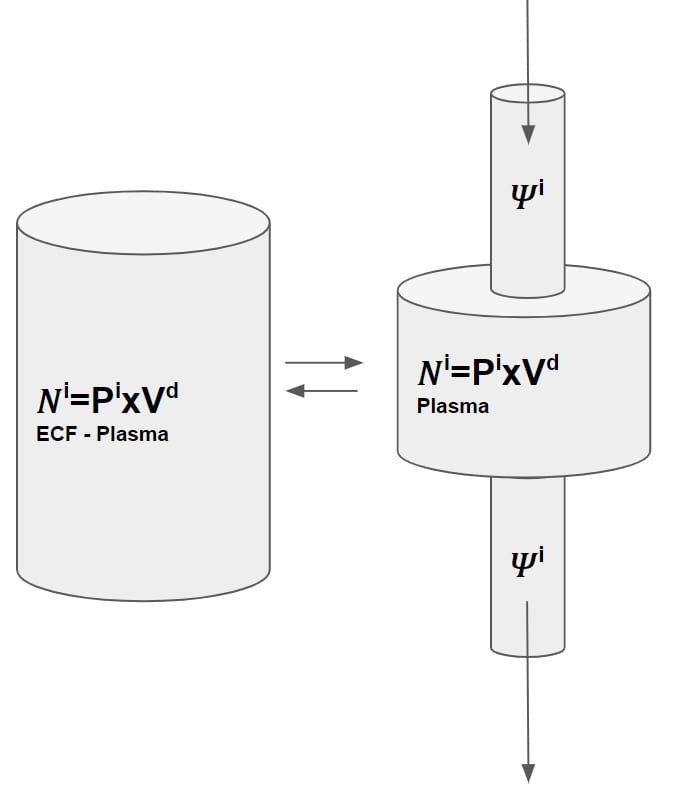
The Inflow
In this diagram, the plasma volume is the big disc in the middle of the right side ensemble. Ψi seems very forbidding but just means the flow into our plasma of something named ‘i’, our old friend from the prior paragraphs. This i could be metabolites (like amino acids) coming in from lunch or leaving cells as proteins degrade. It can be carcinogens coming in from a sausage pizza. Inflow need not be steady in time, it usually is not.
In the drawing you can see an old friend – Pi – but with its little ‘i’ at the top not the bottom. At the bottom the little ‘i’ looks to far from its big letter, so I put the ‘i’ at the top. For the equations, I leave it at the bottom which is conventional.
The Pools
Ni inside the big disk on the right stands for the ‘pool’ of i in plasma. If Pi is its concentration there, and Vd is its the volume i dissolves in, the product Pi x Vd gives the total amount of i in the pool, or Ni.
The cylinder at the left is the total of fluids outside of cells (extra-cellular volume or ECF) minus the plasma volume. Stuff in plasma can equilibrate via the capillaries between the plasma and the non-plasma ECF. For any i, and any amount of dwell time i has in plasma, Vd can range from plasma volume to the whole ECF.
The Outflow
This occurs through the kidneys, into the urine. I have made the symbols for inflow and outflow the same (Ψi), because it is easiest to understand things when inflow and outflow are equal. In the common situation, between meals, they well may be. But what I have to say now does not depend on inflow matching outflow.
The Ruling Equation
When Inflow Matches Outflow
In the steady state, where Ni is constant and inflow matches outflow, the mean life – dwell time – of i in Ni, called ti, is the ratio of Ni to Ψi:
Eq. 2: ti= Ni/Ψi
I cannot derive this equation here. That would involve us in what I did 50 years ago. Those of you with a grasp of calculus can derive it as I did. Note that I have put the i back at the bottom because for equations that is usual, and prettier.
Equation 2 states something obvious if you think about it. When the traffic of a molecule through the ECF is high relative to the pool size of the molecule in the ECF, molecules have a good chance of leaving faster than when the traffic through is lower. Think about a mill dam along a river. The faster the river flow for the volume of the mill pool, the clearer the water – of silt, of leaves, of toxic runoff.
When We Eat, Inflow Does Not Match Outflow
Equation 2 will hold not only when inflow matches outflow, but when inflow is changing and outflow also changing in response. The only difference is that in any one specific interval of time Ni and Ψi are at their mean values for that interval*.
So one can track changes in Ni as a function of changing Ψi and expect to find ti remains constant.
- *For those who like this kind of thing: The distribution of entry rates is convoluted on the function for outflow rates. That latter is always exponential with time because loss rate is proportional to plasma concentrations giving the first order differential equation whose solution must include the kinetic term of the form e-T/ti where T is time. One can derive the non-steady state version of Eq. 2 for a general entry distribution h(t). The general equations lead directly to common isotope kinetic measurements. Although I reference my own paper, it makes no claim for uniqueness, and anyone competent in use of calculus can derive as I did.
Translation of Equation 2 to Kidneys
Equation 2 gives rise to clearance if we just put into it the definitions we already have:
Eq. 3: Ni = Pi x Vd
The pool of any one molecule – number of molecules if you like, is the product of the concentration and the volume holding them. This repeats what I have already said.
Eq. 4: Ψi= Ui x Vu
Urine losses are the product of Ui,urine concentration, and Vu flow rate of urine. This is the simple definition of urine loss rate.
If we divide equation 3 by equation 4 we get mean life as defined in Equation 2,
Eq. 5: ti= Pi x Vd / Ui x Vu = Ni /Ψi
Simply gathering the urine and plasma terms together on the left side, we get:
Eq. 6: Ui x Vu / Pi = Vd / ti .
The left hand term is obviously the clearance of i as derived from Eq. 1b, so equation 6 links traditional clearance, Ci, to Vd / ti, the ratio of the pool size of i to its mean life. Both have the same units, volume/time. Combining equations 1b and 6,
Eq. 7: Ci = Vd / ti; therefore,
Eq. 8: ti = Vd / Ci
In other words, the ratio of the volume of distribution of something (volume) to its conservation clearance (volume/time) is the kinetic turnover constant, its mean life (time).
Rearranging the basic clearance formula of Eq 1b: Pi = (Ui x Vu) / Ci.
If we multiply both sides of Eq. 8 by Pi:
Eq. 9: Pi x ti = [(Ui x Vu) / Ci ] x [Vd / Ci ]= Ci–2 (Ui x Vu) x Vd,
so for any given urine excretion of i and volume of distribution of i the product Pi x ti is the inverse square of clearance.
Because this product, Pi x ti , is the exposure of cells to i, the mean concentration times the mean time it is present as clearance falls, cell exposure rises at an accelerated rate.
Why Are Clearance and Mean Life Linked This Way?
In one sense I could say they simply are, and mathematics, being a good language, has made reality clear. But that is unhelpful, as we could do better with some imagery.
From equation 1b, you can see that clearance is the ratio of the plasma traffic (urine concentration times urine flow) to plasma concentration.
As clearance rises, for example, plasma concentration will fall for any inflow – and therefore outflow – of a material (its plasma traffic). That will reduce the pool size for that material in plasma and the ECF, and therefore lower mean life which is the ratio of traffic to the total size of the pool. So clearance essentially relates the concentration of a molecule to its movement through a volume of distribution, and that, in turn, sets mean life for the molecule in that volume.
An Example
We Eat a Toxin with Lunch and Dinner
Just to show how this works, let’s consider a toxin in our food, and that we eat it sometimes. On the day of interest we eat 4 mmol of it, split between lunch and supper. It could be pesticide in our veggies and fruits, or carcinogens in our deli. I am making this up as an illustration, but the numbers are not unrealistic.
Clearance Sets Average Plasma Concentration and Mean Life
Conservation Clearance
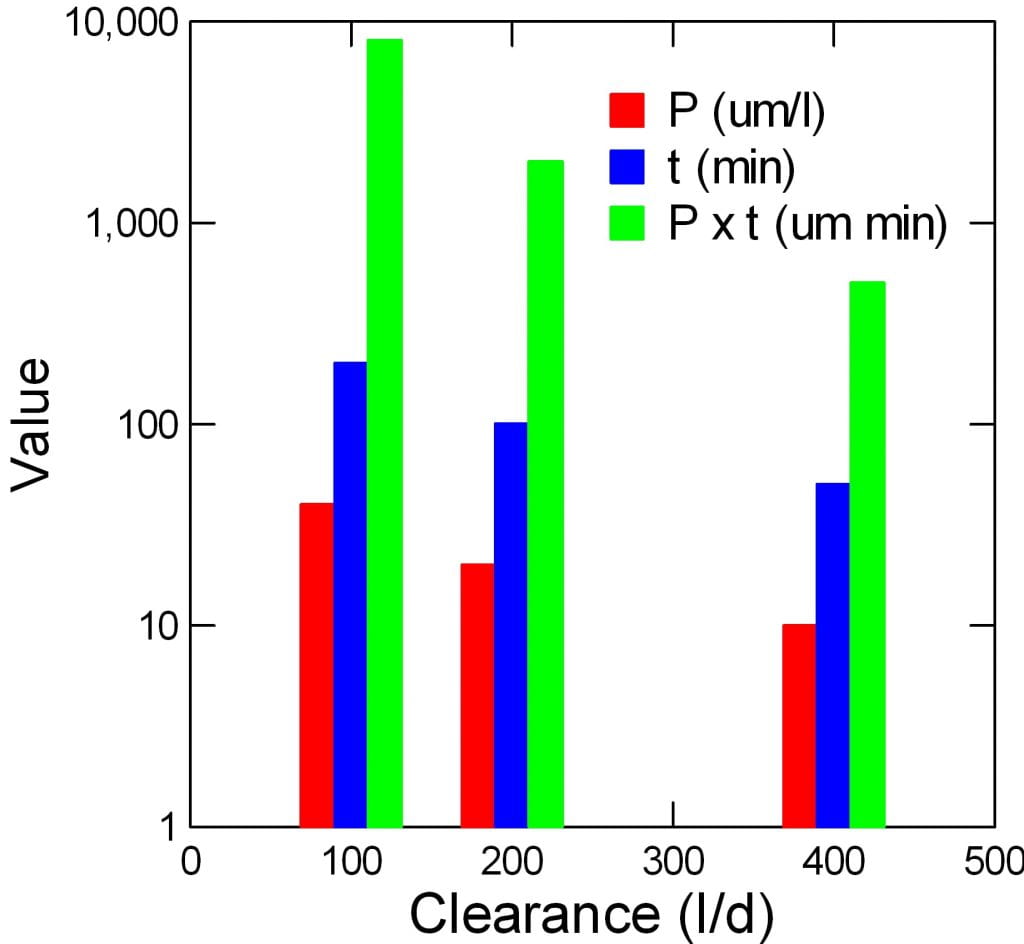
The stuff is removed by filtration and also secreted by our renal cells – they do this with lots of toxins. The clearance is therefore higher than glomerular filtration: 400 liter/day (278 ml/min). Using this daily clearance, and assuming all of the 4 mmol was excreted in the day, the plasma average concentration is simply 4 mmol/d / 400 l/d or 10 umol/l.
Mean life, given an assumed extracellular fluid volume of 14 liters (plasma and non plasma ECF), and that the toxin equilibrated into that total fluid volume, would be 14 l /400 l/d or 50.4 minutes (1440 minutes/d).
The average concentration multiplied by the mean time of cell exposure to the toxin is 50.4 minutes x 10 um/l or 504 umol min. By ‘cell’ I mean the body cell mass perfused by ECF, and of course this includes the kidney cells. These latter have special exposure because they secrete the stuff.
On the graph, I have plotted these numbers as bars above the 400l/d clearance. I logged the vertical axis so you can see so wide a range of numbers all on one graph.
Clearance Falls by Half
The bars over clearance of 200 l/d show what happens. Both mean life (blue) and plasma mean concentration (red) double, so the concentration x time exposure (green) rises by fourfold.
A fall in clearance could be from loss of overall renal function, which means loss of GFR. It could be because some other molecule, like a drug or even a food metabolite, reduced the secretion rate. It could be both.
Clearance Falls by 75%
When toxin clearance falls to 100 l/d, mean plasma levels and mean life both rise fourfold above the original 400 l/d condition, and their product, the concentration time effect rises 16 fold.
Of course this is mere illustration, but it shows how clearance, by affecting both average plasma concentrations for a given traffic through the body and also the kinetic component (how long it takes to remove something that is transient) can create very large effects for the body cells.
You Need Not Be a Toxin
I have chosen a toxin, but the very same reasoning applies to all molecules that transit in waves – a bolus removed over time as opposed to steady production and loss. For the steady production – steady excretion condition, mean life has little practical significance. But so many things come in over a brief part of a day and are eliminated in waves of urine loss that mean life will affect what cells make of them – use, damage, hormone action, for examples.
Mean Life and Clearance are Intrinsic to Kidneys Within the Systemic Setting
It is easy to pass by mean life when one speaks of clearance, and view clearance as just a way of expressing an obvious principle of conservation.
But when the kidney is seen as part of an open systemic system, with food and fluids coming in at irregular intervals and their wastes removed likewise over times thereafter, mean life arises as an natural property of the system.
Likewise, what we call ‘clearance’ arises as a necessary property of the system altogether apart from conservation. In fact, clearance, the volume of distribution, and mean life can be thought of as independent determinants of the system in that any two of the three define the third.
The confusions, if any, arise simply from an undue focus on the body during steady state conditions such as fasting. Then, these dynamical properties lie dormant and simple conservation provides all we need to know. But because we do indeed eat and drink, most of life is spent in non steady state conditions when dynamic properties can affect concentration x time effects.
Mean Life May Help Understand Why Clearance is So High
Many have noted how a bare fraction of normal GFR sustains life, and a fall of even 50% gives no obvious symptoms. Yet, such a fall is undesirable as a careful study variably reveals abnormalities in hemoglobin level, mineral metabolism, and increased risks for cardiovascular disease.
Perhaps because concentration x time effects rise as the inverse square of clearance, we require the ‘extra’ clearance, above what we need to feel well. Even though adjustments of tubule reabsorption may keep many plasma factors normal, the concentration x time effects of toxic molecules we eat or produce – as an example – rise as the inverse square of the fall in clearance. We do not measure secretory rates in medical practice, only GFR, and secretion may fall independent of GFR.
In the search for ‘uremic toxins’, meaning molecular mediators of the obvious impairments that arise from even modest reduction in conservation based filtration rate, it may well be that dynamical measurements may yield more insights than static ones. Metabolic and food toxins that come and go in waves over the food cycle can fail to ‘correlate’ with systemic disease arising from reduced filtration, if all we have is their fasting levels.
Relevancy to Stone Formers
Just because they do have, on average, modest reductions in conservation based clearance, this large population is a special interest. Millions of people, rather on the younger side, may harbor unsuspected increases in food or food metabolism derived toxins whose concentration x time values are much above normal. The idea sounds perhaps novel, but the math is simple.
This matter is specially important because cardiovascular disease risk appears to rise, especially among women, rather early in life.
The Tyranny of Fasting Values
Imagine, given all this, how inadequate serum measurements may be for gauging renal contribution to health.
Of clinical value, of course. We have built on them the palace of clinical nephrology.
Even so, a universe of opportunity remains unexplored. Quite possibly fasting values too much limit our gaze. This is, of course, mere conjecture.
I want to thank Dr John Asplin for reading this article and checking over the mathematics. He is my invaluable critic, thoughtful and exact.

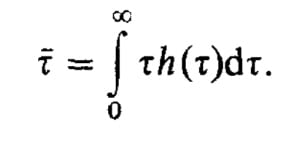
Great article. While the progress and improvement of the different type of kidney surgery over the last 50 years are remarkable. The preventive and testing solutions to avoid kidney stone are not so good. I personally admire the great urologists who saved and prolonged many life. I’m not so happy about the archaic way of testing urine, blood and whatever else is necessary to avoid kidney stones.
Hi Jacques, Thanks for reading it. You are in a select band. As for the archaic, I am not utterly sure. I suspect stones occur because clusters of people are genetically unable to tolerate the diet we all eat, especially its high sodium, low potassium, low calcium, and high refined sugar content. The 24 hour urine is the only available measure of diet intake- questionnaires are less accurate and plenty cumbersome. A slick new molecule might help reduce stones, but not be as good for stone patients who have increased risks for hypertension, and both cardiovascular and bone disease. I vote for a better diet. Regards, Fred
Thank you Dr Coe. You’ are 100% correct. It is time for me to change my diet. I’ve been resisting for years.
The details/math are too complicated for lay person ‘s purpose. A summary would be helpful: such as to increase the clearance, will increasing liquid volume & output be helpful?
Hi Lute, I know, and I said so in the article. My summary was at the bottom: because clearance has several effects, even small losses of kidney function may have bad effects on people, so stone prevention is very important as is care during surgery. Thanks for taking a crack at the article – few will, but I hope physicians and scientists may. Regards, Fred
Hi Dr. Coe,
Thank you for another great chapter and for making this topic accessible to patients!
My trusted nephrologist has told me that my kidneys are healthy, so that isn’t an issue. However, now I’m interested in learning how to interpret test results to see whether or not I have what you describe as a modest decrease in kidney function or not.
I have multiple renal function panels and 24 hr urine tests to work with. But there seem to be unknowns and confounding factors, and no well-defined “normal ranges.”
Do you have any suggestions on how one could use renal function panels and 24 hr urine tests results, perhaps together, to get a good answer to the question of whether kidney function is above average, normal like a non-stone former, or just adequate? Thanks.
Al
Hi Al, for clinical purposes, the eGFR is calculated from serum creatinine and kidney function is classified into five groups from eGFR and other measurements. All physicians have been trained to use the system, and it is the only one acceptable for clinical practice. I have not detailed it on my site as it is derivative to our stone focus. The 24 hour urines and serum creatinine levels common to stone work give exact measures of creatinine clearance, but it can exceed glomerular filtration because of creatinine secretion, and is therefore not used in conventional renal medicine practice. I recognize I am skirting the real issues here, but want to avoid too deep an entry into the chronic kidney disease (CKD) area, it being complex and perhaps a bit contentious, and my being rather specialized in kidney stone prevention. My articles on reduced kidney function in stone formers all used papers based on the five CKD classes. Regards, Fred Coe
Hyy could u pls explain more on what renal clearance is performed
Hi Fiyinfoluwa, It is measured using blood and urine measurements of a marker like creatinine, and gives a measure of kidney function. If creatinine excretion in a timed sample is x mg/hour, that value divided by the plasma concentration of creatinine in mg/ml will give ml/hour of clearance. Regards, Fred Coe
Hi Dr. Coe! As a former patient of yours, I’m delighted to report that I have been stone free since meeting with you nearly 8 years ago. I continue to do check ups with my local nephrologist and one area of concern that I still have, is my creatinine level is still high at 1.5 and eGFR is low at 55. Otherwise, all of my other numbers look great and I’m working with Jill on diet. My last 24 hr urine collection was excellent and I’m working to maintain that. Jill and my nephrologist both wondered if I possibly suffered kidney damage due to prior stones. Not sure what other causes could be behind these two numbers being out of range. At 49 years old and otherwise great health, I’m still searching for answers. Any help or thoughts on this would be greatly appreciated!
Warm regards,
Steve
Hi Steve, I am so glad for the excellent outcome. The high creatinine and reduced eGFR, is there increase of urine protein? Is your urologist sure that drainage is normal? Is there loss of kidney mass from prior stones? Loss of mass is easily detected and might have occurred from obstruction. It is not prone to progress if drainage is normal. Isotope renograms are my favorite to be sure about drainage – my first publications were about their mathematics. Best, Fred
Thank you, Dr. Coe! You’ve given me a few good questions that I can follow up with my local physician. I am unsure on both the increase of urine protein and whether or not drainage is normal. I did just have another blood test and my creatinine came down to 1.39 and eGFR up to 59. Could this improvement be based on my increased fluid intake and better diet? Is it possible to continue improving? I’m certainly hoping so and working toward that end.
Thanks again for taking the time to comment…and for your site introducing me to Jill Harris. She is wonderful!!!
Regards,
Steve
Hi Steven, Getting better is good, and let’s hope that continues. Be sure about drainage, and that the protein is from bleeding not the kidneys. Regards, Fred Coe
Hello Dr. Coe.
Thank you for sharing your brilliance and expertise with so many! It is very much appreciated.
May I ask specifically about my elevated pH on my Litholink results?
My Nephrologist “assumes” I’m a calcium oxalate maker.
With the pH from two 24-hour urine collections being elevated (7.022 & 7.044), I’m wondering if calcium phosphate stones are a more accurate diagnosis.
My oxalate levels were 31 and 41.
As a side note, I have Membranous Glomerulonephritis, CKD #3, and hydronephrosis, in addition to two stones, 4 mm and 5 mm.
Thank you kindly!
HR
Hi H.R. The high pH is more likely associated with calcium phosphate stones, but stone analysis is the only sure way to know their type. I must say at pH 7 CaP is rather likely. Your oxalate level at 41 is substantial, and there is no reason not to lower it by increasing diet calcium to 800 or 1000 mg/d from food sources. Your hydronephrosis could reduce renal function, so every effort should be made to assure normal drainage. The membranous nephritis seems an additional and unrelated problem with its own treatment issues. Incidentally, with hydronephrosis and high urine pH, be sure your 24 hour urine ammonia is measured and is not high – it is possible to be infected with bacteria that produce ammonia from urea, raise urine pH, and cause struvite stones. They have nothing to do with oxalate. Regards, Fred Coe