The title accurately reflects the pragmatic value of the new research I review here. This work shows, for the first time, how one can use urine supersaturation measurements as an estimate of kidney stone risk. It also tests more rigorously than any study to date the urine supersaturation hypothesis that places supersaturation in a position of high primacy in kidney stone formation.
That test supports the primacy of supersaturation, and at the same time shows us how to use supersaturation as a graded risk factor, in the same way we use urine calcium, oxalate, citrate, and volume.
I wish to thank Drs Gary Curhan (Harvard) and John Asplin (Litholink) for their careful review and corrections to this article.
The beautiful thermodynamic partial differential equations expressing free energy changes with phase change comes from Phase separation of polymer mixtures induced by light and heat: A comparative study by light scattering, Advances in Natural Sciences: Nanoscience and Nanotechnology,
The Urine Supersaturation Hypothesis
What Is the Hypothesis?
As far as a crystal is concerned, all of the established urine risk factors for stones act through supersaturation. A crystal cannot respond to calcium, oxalate, or urine volume directly, but only to the free energy produced by the calcium oxalate or calcium phosphate free ion product in relation to the solubility product – the supersaturation.
Given this, we generally base our practice and research upon the hypothesis that urine supersaturation is the efficient cause of stones, the force that compacts solution ions into crystal lattices. This has led to the clinical dictum first formulated by Dr John Asplin: ‘In someone who is producing new stones, urine supersaturations are too high with respect to the crystals forming. Therefore, whatever the supersaturation under that circumstance, lower it.
Supersaturation Does Not Act Alone
Anchored Sites
Of course urine supersaturation acts within a complex system. Randall’s plaque offers an anchored surface for nucleation of new stones, so urine supersaturation is not necessarily needed for de novo nucleation so much as growth of crystal on a preformed template. LIkewise, tubule plugs, almost always apatite, present their open ends to the urine and urine supersaturation acts to grow new crystals on that preformed surface.
Citrate
Citrate, the fourth established urine risk factor, exemplifies another kind of mechanism. Citrate can directly attack the growth surface of calcium crystals, and interpose itself so as to disrupt growth and even force dissolution – a so called ‘crystal inhibitor‘. Citrate also acts on supersaturation, by binding calcium in a soluble complex so less is free to bind with oxalate or phosphate. Likewise, calcium bound citrate is not free to attack crystals. So the calcium – citrate ratio can be of importance in determining stone formation.
Macromolecular Crystallization Modifiers
Urine contains about 1800 individual proteins/peptides many of which attach to crystal surfaces and affect further nucleation, or growth, and can act as a matrix to bind crystals into stones – macromolecular inhibitors. Genetic or other modifications of these many large molecules could condition whether stones form, and much research has concerned the issue.
Anatomy
Like any free energy, supersaturation waits for opportunity to dissipate itself, in our case via phase transformation. In fact, it is no doubt citrate and the large molecule inhibitors that permit urine to maintain supersaturations over many days, as they do. So any drainage problems that raise the mean life of an element of urine within the kidney are apt to result in local dissipative crystallization. Medullary sponge kidney is a likely example of so called ‘stasis’ crystallization. Likewise for calyceal diverticula, and the common stones that form in bladders behind outflow obstructions.
Prior Tests of the Supersaturation Hypothesis
Given all of the factors that stand between supersaturation and its final expression in phase transformation – stones, empirical testing of the urine supersaturation hypothesis matters greatly. No one can quibble about what supersaturation is, or whether it is the necessary force for crystallization. But quibbles are plentiful when one says that urine supersaturation actually does express itself as a risk factor for stones, given the complexity of the renal and urinary systems. Against those quibbles, past scientists have placed their motes of positive argument.
Stone Crystals Match Urine Supersaturation
We have shown that stone crystal composition follows supersaturation. No doubt but that supersaturation is acting here as an agent of crystal formation. But this correspondence could be tested only for the pH sensitive calcium phosphate and uric acid phases. Had the observations failed to show matching, the hypothesis would have been rejected, so the data are not trivial. But the approach fails for calcium oxalate crystals that are insensitive to urine pH.
Use of Trial Data
In prospective trials, one can ask if reduced stones correlate with reduced supersaturations. In one diet trial, by Borghi, and one trial of hydration, I have pointed out that the active treatment patients who formed fewer stones had lower urine supersaturations.
The authors of a new study obtained the full data set from the abovementioned Borghi diet trial and could associate initial supersaturations and treatment changes in supersaturation from baseline with new stone outcome in the active (low sodium high calcium) arm. They were able to extract a quantitative relationship between change in supersaturation with respect to calcium oxalate and new stones.
This use of the trial data could not have falsified the supersaturation hypothesis. Within the original trial, the low sodium high calcium group formed fewer stones than did its control diet group, and in keeping with the supersaturation hypothesis had the lower urine supersaturation. Failure to find a smooth correlation to predict new stones from baseline supersaturations could have reflected simply variabilities that the original trial design did not seek to minimize. Therefore, that the results of the study supported the supersaturation hypothesis is not of crucial importance.
Case Control Risk Factor Evidence
The Curhan group at Harvard has tested the hypothesis that urine calcium, oxalate, citrate, volume, and other characteristics are causes of stones using a powerful epidemiological design.
Three cohorts, two of them female (red in the figure below) and one male (blue) contributed large amounts of information to investigators at Harvard over decades of life. Included in this was kidney stone onset. From a well chosen subset of people who formed stones and people who did not form stones, the Curhan group collected 24 hour urine samples submitted for comprehensive assessment of the usually recognized risk factors and the additional data needed to calculate supersaturation.
For each, the tests were two: Do the stone formers differ from the controls? Does the relative risk of becoming a stone former increase with the magnitude of the proposed risk factor – dose effect?
In each case, this group was testing hypotheses that the factor was a cause of stones. Failure to find an effect in their case control design would be powerfully against the hypothesis in question.
Now, the same group has provided us equivalent data concerning the supersaturations.
Graphing Conventions Used Here
The top of each bar in the graph below is the relative risk within a given urine risk factor grouping (for example, a range of urine calcium excretions in the upper left panel) compared to a reference range (for calcium, less than 100 mg/day). The bottom of each bar, visually emphasized by the solid filled bars, is the lower 95th percentile of the relative risk.
When the lower 95th percentile fails to cross 1 (the dashed horizontal line), the probability that risk is not increased is below 5%.
For protective urine measurements, urine citrate and volume, the process is simply reversed: The mean relative risk compared to the highest urine value is plotted downward, and the top 95th percentile upward from that. When that top 95th percentile of risk lies below 1, the likelihood that risk is not reduced is below 5%.
In each graph below, the two female cohorts are in red, the one male cohort in blue.
Prior Established Graded Risk Factors
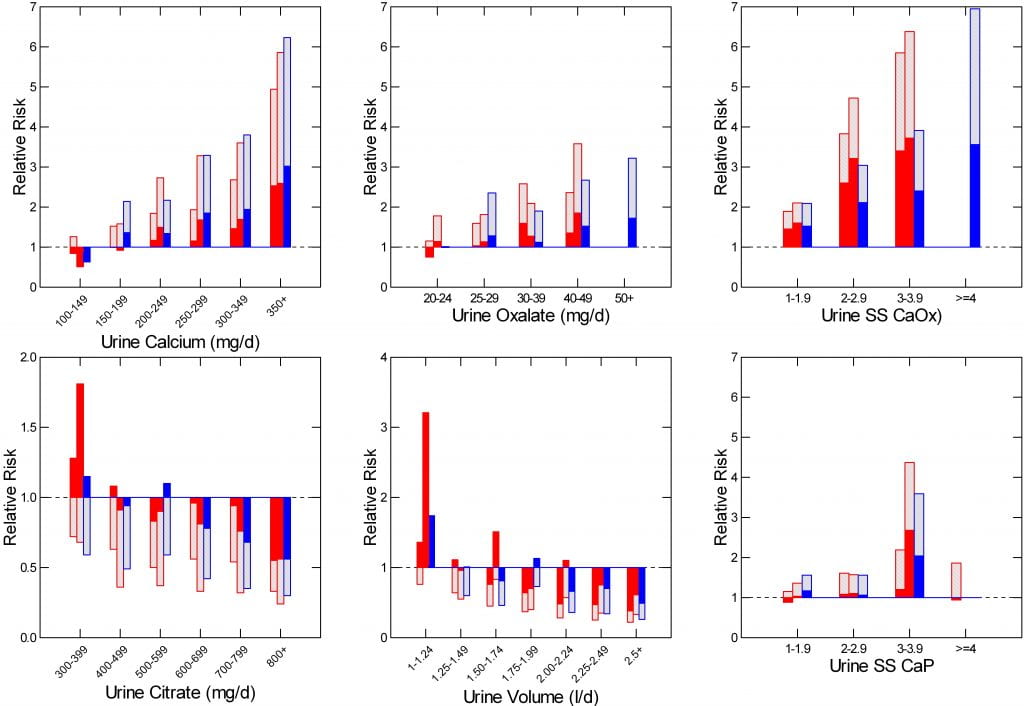
The most important prior outcome of the the entire work has been to prove that four urine measurements satisfy the predictions: Differs between stone formers vs. controls, and shows a dose effect. Urine calcium, oxalate, citrate, and volume – the four panels at the left and center of the figure – show both characteristics.
This work has replaced older and mainly incorrect cutpoints with real information linking a particular measurement to relative risk.
Effects of Supersaturation
Dr Megan Prochaska, in the Curhan group and using the same three cohorts, has provided us with crucial tests concerning stone incidence and urine supersaturations. I have graphed her new findings as in the other four risk factors, using the right hand panels of the figure.
Supersaturation Calculation
Everywhere else on this site, and in most publications, supersaturations are calculated from urine free ion products and established solubility products using programs such as EQUIL – 2. This work differs in that the free ion activity product from EQUIL – 2 is divided by the mean value derived from a group of normal non stone forming controls. This creates a ratio of the calculated calcium oxalate and calcium phosphate supersaturations from EQUIL-2 to the corresponding means of the normals, and that ratio is called the ‘relative supersaturation ratio (RSS)’. Because the transformation of supersaturation to RSS involves only multiplication by a single number (the reciprocals of the means for calcium oxalate and calcium phosphate, respectively) it can only alter the magnitude of the numbers, but not their relative positions one to another. So risk in relation to RSS or to supersaturation will be the same.
Calcium Oxalate Supersaturation
Compared to kidney stone rates in people whose urine had calcium oxalate RSS<1, risk of stones was already significantly increased in the cohorts with urine calcium oxalate RSS between 1 and 1.9 (upper right panel). Thereafter, risk increases with RSS in a progressive manner with p values for trend analysis of <0.001 in all three cohorts. Since the bottoms of the bars do not cross 1 in even the lowest RSS range, risk is almost certainly increased at any RSS above 1.
Calcium Phosphate Supersaturation
For Calcium phosphate (lower right panel), the same, except that risk is not certain in the lowest RSS group for one of the three cohorts. Also, only one of the cohorts provided sufficient data for analysis in the highest RSS group. Even so, trend p values were <0.001 for all three cohorts.
Uric Acid Supersaturation
Dr Prochaska provided data for uric acid RSS which showed progressive stone risk for two of the three cohorts, but I did not graph it. Uric acid chemistry is such that crystallization is rapid as urine pH falls, and treatment is aimed at urine pH. The group does not have stone analysis data, and uric acid stones could therefore not reliably be separated from calcium stones.
Orders of Magnitude
I have made the calcium, oxalate, and two supersaturation scales identical, from 0-7, so as to compare the four risk factors. CaOx RSS and urine calcium match well, the range of risk for oxalate and CaP supersaturation is much smaller, though all four are progressive.
Importance of the New Findings
Critical Test of the Supersaturation Hypothesis
The obvious importance is that this stringent test of the supersaturation hypothesis is not negative. Had it proven negative, one would have had to radically revise our thinking. Perhaps Karl Popper would have been happy with such an outcome, perhaps I might have been, too, but it is easier to have a positive result.
Risk Begins at Modest Calcium Oxalate Supersaturations
The Special Supersaturations Available Here
The RSS values we have for the new work arise from decisions made by Professor Charles Pak. Charles wished to construct a visually clear representation of calcium oxalate, calcium phosphate and other supersaturations on a single graph, and achieved that aim by scaling each supersaturation by the mean of the corresponding supersaturation found in normal – non stone forming – people. The resulting RSS values for each supersaturation therefore run in multiples of a normal population and can fit into a single graph range.
For calcium oxalate, his normals had a mean calcium oxalate ion activity product of 7.3×10-9 M2, the core measure from which supersaturation derives. The solubility activity product is 2.2 x 10-9 M2. This means that his normals had a supersaturation of 7.3/2.2 or 3.31. In the scale Prochaska had available, therefore, the first supersaturation range ran from 3.3 to 6.3 (3.3 x 1.9). Risk was first evident therefore within this range. If we are using standard supersaturations for our clinical work we therefore can approximate that risk begins at above 3.3 and rises thereafter exactly in proportion to her graph.
For calcium phosphate as brushite, the Pak normals had a mean value of 2.35 x 10-7 M2. In EQUIL the solubility product for brushite is almost identical: 2.35 x 10-7 M2. So the RSS and SS values for brushite are the same, and risk from calcium phosphate supersaturation begins above 1.
I wish to specially thank Dr John Asplin for providing the two equilibrium solubility products used above which he obtained from EQUIL and from a difficult to obtain book: Urinary Calculi LC Delatte, A rapadp, A Hodgkinson eds, 1973.
Upper Limit of Metastability Can Mislead Us
Brilliant work by Dr Charles Pak showed us long ago that for calcium oxalate one needs for crystallization to raise supersaturation above an empirical upper limit – the so called upper limit of metastable supersaturation (ULM). Among others, I have explored the significance of the ULM by testing its ability to distinguish between stone formers and well matched controls.
Among both men and women (references to primary publications in the above linked article), I found the critical difference between the measured calcium oxalate ULM and the actual urine supersaturation for calcium oxalate was not impressively different between patients and controls. Likewise I could not find important differences between patients and controls for urine supersaturation. But my two small trials may have lacked power.
Given that calcium oxalate stones may well form anchored to plaque or perhaps to tubule plugs, and given that plaque and plugs provide initial crystal substrate that abrogates the ULM altogether, it may well be that very modest calcium oxalate supersaturation is enough in those who form more abundant plaque or plugs and thereby become stone formers.
Likewise, modern work has revealed that calcium phosphate may play a special role in calcium oxalate stones by acting as a link between plaque and perhaps open plug ends and the initial calcium oxalate crystal formation.
Supersaturation is a Progressive Risk Factor
The progressive nature of the calcium oxalate supersaturation is compatible with growth on plaque or plugs. This is because even if nucleation in solution no longer matters, there being an anchored substrate, one needs supersaturation to grow the calcium oxalate phase, so growth – and manifest stone risk – will be proportional to supersaturation as in the new data.
Risk Begins at Any Supersaturation for Calcium Phosphate
Originating Species
With the exception of one cohort (females in the lower right hand panel of the figure shown above), any CaP supersaturation raises stone risk, and this is also compatible with what we know otherwise. Interstitial plaque is not affected by urine concentrations, but plaque exposed to urine first overgrows with calcium phosphates of urine origin. Thereafter, calcium oxalate nucleates over the initial calcium phosphate layers. Plugs are almost always calcium phosphate as apatite, so they require some CaP supersaturation.
Brushite
Brushite is a special case that amplifies this peculiar role for calcium phosphate in calcium oxalate stone disease.
Pak showed clearly that brushite is the first crystal phase to form in urine as one raises supersaturations, and this crystal may be very important in nucleation of calcium oxalate. Oxalate in solution can pirate calcium off of the brushite lattice, cannibalizing brushite to make itself – at least in vitro. We measure CaP supersaturation as brushite, the most soluble form of calcium phosphate regularly found in urine. Even slight supersaturation can be enough to nucleate brushite, creating a possible source of high calcium concentration to foster calcium oxalate.
Any CaP SS may be Enough
Altogether CaP supersaturation is like the match more than the tinder, so any at all is very likely enough for calcium oxalate stone formation. In order for calcium phosphate to predominate as the main stone type, one needs higher CaP supersaturation. Possibly, the progressive nature of CaP supersaturation results from people whose stones had higher CaP mineral contents; we cannot know.
Clinical Meaning
Because of what it can do, most of us work to lower CaP supersaturation below 1 in every case we can. These new data encourage us in that practice and add some experimental heft to the proposition that CaP supersaturation may be generally important, even for the common CaOx stones.
But perhaps more importance is that the new data confer on patients and physicians a new fluency and sophistication in the use of calcium oxalate supersaturation. Just as we have abandoned clinical cut point definitions such as ‘hypercalciuria’, ‘hyperoxaluria’, ‘hypocitraturia’ and ‘low urine volume’ as diagnoses, and replaced them by using actual measured values to assess potential stone risk, we can do the same with supersaturations.
A value, for example, of 2 is a lot less risky than a value of 6, for calcium oxalate. This makes supersaturation more like blood pressure, and other graded risk factors, and allows us to stop using such phrases as ‘high supersaturation’ instead of the more nuanced statistical risk the supersaturation implies. Given the 3.3 multiplier to convert RSS to the commonly used SS, the key SS values for clinicians are (rounded): below 3.3 (lowest risk); 3.3 – <6.6, increased risk is present; 6.6 – <9.9, medium risk; 9.9 – 13.1, high risk; >13.1 very high risk.
These values can be used to amplify the Asplin dictum. If stones are forming, supersaturation with respect to the crystals in the stones formed are too high and should be lowered to below the Prochaska delimiters of 3.3 for calcium oxalate and 1, of course, for calcium phosphate.
This is a Major Contribution
The new work provides a critical test of the supersaturation hypothesis. The positive result means we can continue to use that hypothesis as an integral part of our working theory of stone pathogenesis and treatment. For clinicians, the present result offers a clear message: Measure supersaturation, lower it: Aim below 3.3 for calcium oxalate, and 1 for calcium phosphate.


What does this mean to kidney stone formers in plain language? Should we increase our citrate intake via citrus juice in water, etc? How is this information going to help me right now in my home?
Thanks – I enjoy your comments, even though many writings require much computer searching to clarify some of the scientific/math parts.
Hi Yvette, It means that your supersaturation ceases to impose increased stone risk for calcium oxalate when below 3.3, and for calcium phosphate when below 1. You can use these goals to guide your treatment. Regards, Fred Coe
Dr. Coe, I’ve been a member of Jill’s FB page for 16 months after passing two ca ox stones. I have employed every strategy I learned in her course and just received the best 24 hr urine results yet. I’ve been labeled IH, but I did manage to bring my calcium down to 260 from 400. One of my strategies is to drink 1 liter of Crystal Light a day along with following the guidelines for added sugars, animal protein, sodium, calcium, and oxalates. My avg volume is 3.5-4L/day. My SS CaOx was 2.70. My ox was 24. Citrate was 946. SS CaP was 1.11. My pH was high at 6.841, but I’m sure it’s because of all the citrate and the report stated I wasn’t at risk for CaP stones if I keep my volume up. The SS Ua was .04 and the UA was .384. Na, K, Mg, P, Nh24, Cl, Sul, UUN, PCR, Cr, Cr 24, and Ca 24 were all in the green as low risk. The Ca24/Cr24 was 250.
My question is:
Given that all my results are low risk with exception of the calcium, is it reasonable to suggest to my doctor that I forego the use of thiazide for now and continue with my dietary and fluid strategies and continued monitoring through 24 hr urines? Committing to a lifelong diuretic is something I’d rather do as a last resort.
Dr. Coe, I appreciate so very much your dedication to your work and Jill’s knowledge, support and compassion for each of us. I feel incredibly blessed to have met you both on my journey.
Thank you for your input,
Melissa Howard
Hi Melissa, I think that is reasonable. Of course your physician is ultimately responsible for your care, but perhaps s/he will agree. Regards, Fred Coe
Dear Dr. Fedric Coe .
Thanks for providing in depth knowledge about Stone Disease and its evaluation. I have following queries about 24 hrs Urine Metabolic profile :
Can the 24 urine sample be collected while the caliceal stones are in situ or we have to wait till the stones completely clear
2. Should the patients having bilateral small multiple Caliceal stones be metabolically evaluated ?
3. Impact of calcium supplements on causation of stones and how should the patients on supplements as well as having stones be monitored to maintain the balance
Hi Dr Mehta, The stones in the kidneys make no difference. To me patients with multiple calyceal stones surely need testing and prevention efforts. Calcium supplements can always raise urine calcium and stone risk; lower diet sodium can reduce that risk. I use 24 hour urines liberally to know what to do and see what has happened. Warm regards, Fred
Thank you for reply. Also please let me know after how many days of surgically clearing stones, patient should be asked to go for 24 hrs urine metabolic profile? and why ? Regards..
Hi Doctor Mehta, I wait for about 6 weeks after surgery to be sure patients are back to their normal status in terms of diet, work, and general activity. No data on this, but it seems sensible. Warm regards, Fred
Thanks. I appreciate
Dr Coe,
As a community urologist I love your site. I was fortunate to train with Dr Curhan and have a background in x-ray crystallography so crystal formation is near and dear to my heart.
Typically the strategy I follow to reduce patient risk is to focus on the factor that has highest relative overall risk. Looking at the graphs my understanding is that you normalized for relative risk compared to controls. The scales you use are 1-7 for Ca, Ox, SS Ox, SS CaP, whereas citrate and volume are much smaller.
My first question is does that risk relate across groups? Does a risk factor of 2 mean the same across the groups. Are these all pegged to the same chance of forming a stone?
If it does then managing Ca and Ox give us the biggest return for effort.
If not, then the focus is reducing each factor separately. The question may become how can the relative risk be reduced to below 2 for each factor? Asked this way there seems marginal benefit from getting citrate above 400 or urine volume over 1.25, while a target Ca is under 200 and target Oxalate is under 30.
I’m a little confused by the clinical implication to manage CaP so aggressively. The reported risk for CaP SS is basically flat through to a RSS level of 2.9, while the CaOx SS risk is 2 fold with a RSS over 1.
This would suggest that after your RSS to SS conversion for both CaOx and CaP an SS of 3 has the same 2 fold risk compared to controls for both CaOx and CaP saturations.
Perhaps a unified set of risk profiles could help. Your suggested 5 groups of lowest risk, increased risk, medium risk, high risk and very high risk would need to be comparable across risk factors. This would help to delineate where our clinical time should be spent such as increasing fluid intake vs reducing dietary oxalate.
Thanks once again for your lucid and insightful commentary.
Hi Doctor B, I simply plotted the relative risks from Gary Curhan’s papers. The RR is much smaller for volume and citrate because both reduce risk compared to the comparison groups, so there is only between 0 and 1. For increasing volume RR goes from 1 to below 1. I plotted the lower 95th percentile at the bottom of the bars for those factors that raise risk, and the upper 95th percentile for citrate and volume. My approach was simply that when the 95th percentiles lie above 1 risk is increased. The benefits of raising urine volume above 1.25 and citrate >400 lies in the position of the upper 95th percentiles. I see no a priori reason to choose 2 as a clinical cutpoint, all of these factors are graded risk factors. As I understand the research, any one risk factor is corrected for the others – each confers independent risk vs. the comparison point for that factor. The RSS for CaOx is an artifact of calculation in which SS was divided by the SS of 47 ‘normal subjects’; the SS would therefore scale 3.3 times fold those reported. The problem arose because Gary used Mission Pharmacal testing for some of the SS studies. For CaP SS, there is no such normalization, so the values are SS. Risk is increased between <1 CaP SS and >=1 CaP SS, so one wants to lower CaP SS below 1. Finally, I have hesitated to name risks as medium or high as Curhan did not, and rather prefer his idea of risk as a function of the value of each factor; this leaves to each physician full range of choices for treatment. Regards, Fred
I’m glad I found you informative site. I passed my first small stone at age 63, and 9 months later, I had a 7mm stone stuck in my left distal ureter. I underwent laser lithotripsy and a one week stent.
My 24 hour urine revealed a mildly high Ca. My serum Ca and pth were wnl. I’ve increased my water and crystal light, with a one year f/u. My theory is that my daily steam room visits caused my calcium oxalate stones. I’ve changed this, and have been loosing some weight BMI 28. PMH include GERD, HTN, and Hypercholesterolemia.
Hi Don,
Remember to lower your sodium to under 2,000mg/day to keep your urine calcium lower. Lowering sodium will also help with your hypertension. Keep up the good work!
Best, Jill
Dear Dr. Coe,
I´m a 44 y/o, brazilian nephrologist, working at private practice and as a co-chair of a cooperative Health Plan. Dr Waldir Eduardo Garcia, who passed way years ago, joined post doctoral at your program, he was my teacher at University, our most beloved teacher.
We just don´t have supersaturation to study our patients in Brazil, a 206 millions person nation, even in big laboratories, and that´s my point.
Is there any chance to join a program at Chicago University, thereafter bring the method – and improve the prevention of kidney stones – to our country?
I´m ECFMG certified physician, member of ASN and former mini-fellow of Georgetown University Nephrology program.
Thank you,
Sincerely
Denis Rogerio Aranha da Silva, MD
Hi Doctor da Silva, It is common to lack SS and even comprehensive stone panels in even such advanced countries as yours, and widely in Europe. If you have a cooperative lab in Brazil, we would be happy to work with it to get full scale kidney stone testing there. Because of borders, it is almost impossible to support clinical care in another country with measurements from US, but utterly practical to help lab people in that country produce the required measurements. Please let me know if this is possible. Warmest regards, Fred
Thank you for your support.
Actually we still don´t have a laboratory in your health plan, but parternship with a local one would be a good way to us. Our board of directors are interested in innovations, but they asked about the costs of machines and softwares. I´m going to Kidney Week in november 2019, in Washington DC. May I spend some days in Chicago, around that days, and know your program of kidney stone prevention? That would be a honor to me.
Best wishes.
Denis
Hi Denis, I am sure we would be pleased. Lets consider exact dates, presumably before or after the ASN. Fred
Hi Dr Fred, the ASN meeting will finish in november 10th.
May we consider november 11 – 15 th to me, as an observership?
Unfortunately, I can´t stay many more days in Chicago, my family will go with me.
Our council and physicians are eager about bringing new technologies to our city, and I really believe urine supersaturation will be worth to improve the practice of nephrology herein Brazil.
Thank you.
Denis
Hi Denis, That sounds fine with me. I will write to you by private email, so we can transfer this out of the public space. Regards, Fred Coe
Hi,Dr.Coe
I am so pleasure to see this site, I think you give us a great view and the way of thinking of kidney stone disease. The aim of this website is to make more and more patients away from the pain of kidney stone. it is my job that I want to do, but one things always confuse me , how to get the programs EQUIL – 2? looking forward your responding. cause we found 25% maintain hemodialysis patients are from kidney stone in some area of our country , terrible situation. Thank you very much.
Hello Doctor, Equil 2 was an open source software and none of us has a free copy – long out of date. Those who have it have it embedded in other software. It is bad enough that one of the original programmers wanted to update it and I could not furnish him with a copy to work on. I think it time to do a new program altogether. Sorry, Fred Coe
A Visual Basic translated copy of Equil -2 was found here:
https://billdenney.github.io/equil2/#installation
Hi James, Thanks. John Lieske was once my protege and now runs the stone program at Mayo. I do not know the programmer, but it is a nice thing to do and I will contact him. Best, Fred
Note: Translated to R.
I have cystine stones and I believe I participated in your study about 30 years ago. Do you still see patients? Can you recommend someone? I recently found out that my GFR has been between 43- 50 and have cysts on my kidneys. I tried to visit with a nephrologist in the south suburbs. She said that my GFR should be about 75 for my age. I am 59. I am disappointed that she has not called me with “her plan”. I waited 5 weeks for an appointment and I know that cystine stones are rare, but she should at least tell me to find a different nephrologist. Any help would be appreciated. Thanks!
Hi Mary, I am sorry you were here and somehow got lost. I do indeed see patients, and you can call my clinical coordinator: 773 702 1475. Cystinuria is very complex and we should have done better for you in following up. Just come back, we will have your old records and take up from there. Regards, Fred
After reading much of your site I do not see information about stag horn kidney. Do you address this condition? If not, any recommendations for a thorough study on this condition?
Hi Dolores, I think you mean staghorn stones. If so you are right, I have not written about them. They are a bit special, and their surgery, too. Here is the article about the surgery. Many staghorns are struvite, from infection, some are uric acid, some are just large versions of the calcium oxalate calcium phosphate variety. This article details all the stone types. So, staghorn is not so much a thing of its own as rather a polyglot of other things. If you know the composition that takes you a long way to the right prevention. Regards, Fred Coe
hi Dr Coe
I have a question
is there any standrad reference range for relative supersaturation for calcium oxalate , uric acid and calcium phosphate stones ?
i mean RSS normal reference range
after using Equil2 what will be the range for saying that stone risk is high or low for every type of stones ?
Hi Sal, I answered this on the other article, and this one gives the answer. Risk wises with SS as in the bargraphs showing risk vs. SS level. Fred
Can state Urine sample give approximately same result as 24 hrs collection? Many times patient avoids 24 hrs collection and thus can a single collection useful for treating patient?
Regards
Hi Dr Mehta, I am afraid not. We have found that urine volume, calcium, oxalate, sodium, pH and SS values change during the day and overnight, with meals and activity. Any one sample costs as much to measure as a 24 hour sample and gives a very poor estimate of general risk. I do have a long clinical experience of many thousand patients, and I have been able to convince most to collect 24 hour urines once they understand the value. Regards, Fred
Just had my first kidney stone at age 66. It was a calcium oxalate. A 24 hr urine was not done. As weeks pass I am curious if the PPI I’ve been on for three years may have caused the stone! Any studies indicating it can be a cause?
Hi Cindy
Stones later in life are special. I do not know what PPI refers to, so I cannot answer your question. If you tell me the drug I can help. But as a woman and a bit older, the link may be more informative. Regards, Fred Coe
Dear Dr. Coe,
I had a 24-hour urine (not LithoLink). In the first results posted in my Chart, my calcium was 143mg/24hr range 50-250mg/24h. I was very happy but when I received the details of the SAT24 it listed Calcium, 24hr. U 322H mg/24h<200. Can you please explain the discrepancy in the values. Thank you.
Hi Antoinette, I do not know what SAT24 means but it would seem that how the original lab results were entered into the medical record created an error. I would suggest you ask for the actual original lab report from the vendor which will (by law) be accurate and ignore MyChart as a derivative and probably incorrect rendition. Regards, Fred Coe
Thank you Dr. Coe. If I may rephrase my question. The traditional 24-hour chemistry chemistry performed by the hospital in Toledo, OH showed 143mg/24hr range 50-250mg/24h. The super saturation report from Mayo Clinic Laboratories – from the same 24-hr sample stated Calcium, 24hr. U 322H mg/24h<200. What number is considered more accurate and why the discrepancy?
Hi Antoinette, I cannot be sure but this is what I think. The urine was sent out to Mayo Clinic where measurements were made. The true urine calcium is 322 mg/24 hours. The hospital redacted the number from Mayo Clinic incorrectly as 143 mg/24 hours. The only other possibility that seems right is that you did two 24 hour urine collections, one measured at the hospital as 143 mg/24 hours and another sent to Mayo. Were there two 24 hour urine samples collected? Regards, Fred Coe
No there was only one collection but in 2 jugs. My physicians are going with the the Mayo numbers. Because the number from the hospital came within 48-hours and I waited for a week for Mayo, I was just trying to understand if there was a difference in the testing. Maybe the hospital tested just one of the jugs. Thank you again for your time. Have a good week!
Hello Dr Coe,
I have possible RTA and I have no urine citrate at all with a high urine ph 6.8-7.
Is it possible to ever get a CAP SS under 1 with lowering urine calcium with thiazides and applying all your tools like min 2.5 ltr a day of fluids and reducing sodium intake.
I appreciate your comments thanks again.
Hi, I had suggested you might start with very low diet sodium – 1500 mg – as a safe beginning. Fred
Hello, I am in a master’s program in biotechnology. I’m working with a pathology lab to determine the risk of kidney stones for my final project. I am having trouble finding Ksp values for chemical species found in urine. Do you have any resources you are willing to share? Would you mind exchanging some emails? I’d love to get additional input into my project.
Hi Paige, I believe that in the many urine saturation articles on the site I referenced a few of the older ones that give (within themselves) the actual values in calculating programs like EQUIL. I have had GEMINI perform an in depth search for you which is indeed so excellent I copied it onto DOCS for my own use. Here is the public linkRegards, Fred
Hi Dr. Coe,
I have found your website to be so informative and helpful.
This may seem like a silly question, but is there any way that high uric acid could lead to the formation of calcium oxalate stones?
My husband’s stone analysis showed his stone was 95% calcium oxalate mono hydrate.
However, his 24hr urine analysis showed calcium to be in the normal range (249/ range <250) and his oxalate (results say “oalate”-typo?) normal at 1.05 (range <2.0).
Uric acid results were seemingly contradictory as in one place titled “Uric Acid 24hr Urine” shows high at 810 (range <700) but in another area titled “Uric Acid” it shows in range at 0.26 (range <2).
The only other item out of range is Creatinine at 2057 (range: 800-2000j.
My husband did pass several stones with about 24hrs or urine collection, so not sure if that might have had a bearing on creatinine number.
Again, perhaps a silly question, but do uric acid and/or creatinine have any meaningful associated to his calcium oxalate stone formation?
Thanks so much for your insight.
Hi Jamie, A calcium oxalate monohydrate stone with normal 24 hour stone risk factors usually means urine volume was low – below 1.8 liters/d. I would be looking for hints that was the case in the years prior to the stone onset. Uric acid in amount per day differ from uric acid supersaturation which was low. It is not the problem here. Best, Fred Coe
Also, I forgot to mention that urine pH was fine at 6.7 (range <7)
Also, I apologize for the typos in my original post. (No way to edit after submitting.)
Thanks again!
Hi Jamie, The pH is not at issue. Think about work, sports, travel stress that might have compromized hydration the past few years. Regards, Fred Coe